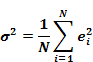
( 1 )
OUCC Proceedings 4 (1966)An Experiment to Estimate the Errors made in taking Compass BearingsH. I. Ralph |
OUCC Home Page |
The error made in taking a bearing between two points has a number of causes,
aggravated under cave conditions, of which the most important are:
1) misplacing of the compass and sighting object
2) intention only to read to a certain accuracy
3) inability to read to an infinite accuracy
4) variations in the magnetic field
The last of these is a systematic error and is not considered here, but the
first three are random errors and are, as such, amenable to statistical
analysis.
The quantity used to denote the likely error is called the standard
deviation and is given the symbol
s.
If a large number, N, of readings are taken, and the error made in the i-th
reading is ei, then the square of the standard deviation ( sometimes
called the variance ) is given by the equation
|
|
( 1 ) |
The second type of error listed above, which will be called the tolerance
error, is the error due to the coarseness of the scale used. For example, the
error made if the scale is read to the nearest 5° may be anything up to 2½°
either way. The tolerance error is equally likely to be any value inside the
tolerance but is certainly not going to be more than the tolerance. This case is
easy to deal with and the standard deviation of the tolerance is calculable
theoretically. If the scale is read to the nearest 5°
the standard deviation turns out to be 1.28°. In general, if the scale used is
marked in divisions spaced at a distance a, then the standard deviation of the
distribution of tolerance errors is a/3.46.
The distribution of the first and third types of error, which will be
called the position and reading errors respectively, are certainly nearly normal
distributions, but it is unnecessary to attribute any particular form to them.
The standard deviations of neither are calculable, so that an experimental
determination of the total standard deviation must be devised that will
distinguish between them. Fortunately, this distinction is facilitated by the
very thing that makes it necessary.
The error made in measuring angles will be the sum of these three errors,
assuming no variation in the magnetic field. In this case the general theory of
stochastic processes gives that the standard deviations add in the squares. That
is to say, if the standard deviation of the position error, the tolerance error,
and the reading error are
sp,
st,
&
sr
respectively, then the standard deviation,
s,
of the total error is given by
![]()
Now
sr
and
st
are independent of the distance, d, between the compass and the sighting object,
but
sp,
the error due to the misplacing of the compass and the sighting object can be
written as a/d where a is some quantity independent of d. Thus
![]()
Since
st
can be calculated, if
s
is measured experimentally for two or more values of d, both
sr
and a may be individually calculated.
Objections can be raised to the simple device of setting up a compass and
sighting object, taking the bearing of the latter from the former many times,
and evaluating
s
from equation ( 1 ) subsequently. Were this done the same reading would be
recorded each time, and, taking the true value of the bearing as the average of
all the readings,
s
would turn out to be zero. This does not point to the failure of equation ( 1 )
but rather to the fact that the errors in such a procedure are not random.
Indeed they are not, each being just the same as the other.
Four points must be borne in mind when devising the experiment to measure
o :
1) the reading recorded in any particular case may be biased by a memory
of previous readings if the same or nearly the same angle is measured twice.
2) it should be certain that the tolerance error really is random in the
way indicated above.
3) it should also be certain that the position error is random, neither
this nor the last error will be random if the compass or the sighting object is
not continually moved during the experiment.
4) equation ( 1 ) is true only after an infinite number of readings have
been taken. An error is introduced because only a finite number readings can be
taken. If the true value of the reading is not known this error enters twice, as
the true value has to be taken as the average of all the readings.
The experiment to be described here takes account of the fourth
difficulty while overcoming the first three. Basically, it comprises measurement
of the internal angles of an n-sided polygon with a compass and comparing their
sum with the known result. The only drawback is that the number of readings that
has to be taken to achieve a given accuracy increases by a factor of 2n.
If there are m polygons each with n sides, and the error in measuring the
sum of the internal angles of the i-th polygon is ei, then the
standard deviation, o, is calculated from the formula
|
|
( 2 ) |
The answer obtained for
s
will be accurate to within 100/
![]() of the actual value ( that value would be obtained if an
infinite number of polygons were used ). These two results are derived in the
appendix.
of the actual value ( that value would be obtained if an
infinite number of polygons were used ). These two results are derived in the
appendix.
Certain form should be observed during the experiment in order to ensure
that reliable answers are obtained. Clearly, since
s
varies with the distance d, all the sides of the polygons should be the same to
within a very few inches. The most suitable polygon to use is a regular
pentagon, in the case of three and four-sided figures there would be noticeable
similarities between the readings and this would detract from the reliability of
the answers. Some order of reading the bearings should be used that introduces
the maximum confusion into the memory of previous readings.
The method of working out the sum of the internal angles is important.
Each angle should be evaluated separately as the difference between the forward
and back bearings at each point, these angles then being summed. If a different
method is used, much of the information from the experiment could be unwittingly
thrown away. This method also minimises the effects of magnetic field variation.
The procedure is then as follows. First stake out a large number of
pentagons with a given length of side and evaluate
s
for that value of d from equation ( 2 ). Then repeat this for at least one more
value of d. From the formula
![]() ,
,
sr
and a may easily be calculated.
st
is given by:
![]() ,
,
where t is the tolerance ( e.g. 1° if readings are taken to the nearest degree).
Appendix
![]() ,
,
If the error on the sum of the internal angles of the i-th polygon is ei,
then
![]() ,
,
so that
![]() ,
,
Define
scalc
and
stcalc
by the equations
![]()
and
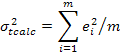
Then
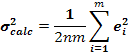
Now the probability density of getting an error ei for the i-th
polygon is Pe( ei ), where
![]()
If fi =
![]() and the distribution
of the fi goes as Pf( fi ) then
and the distribution
of the fi goes as Pf( fi ) then
![]()
It should be noted here that it is not necessary to assume that either the
position error or the reading error follows a normal distribution. The error on
one reading follows a distribution that is certainly not Gaussian but the
distribution of ei is the convolution of ten such distributions which
are all similar so that it is very close to a normal distribution.
The standard deviation of Pf may easily be calculated by the usual
formula and it turns out to be
![]()
![]() ( =
( =
![]() ).
The standard deviation of the sum
).
The standard deviation of the sum
![]() (
(
![]() )
is then clearly
)
is then clearly
![]() so
that the standard deviation of the distribution of the quantity
so
that the standard deviation of the distribution of the quantity
![]() is
is
![]() .
It is now easy to see that the standard deviation of the quantity
scalc
is about
.
It is now easy to see that the standard deviation of the quantity
scalc
is about
![]() .
.
The
![]() .
is the likely error that will be made in measuring
s
if m polygons are used.
.
is the likely error that will be made in measuring
s
if m polygons are used.
H. I. Ralph,
St. John’s College,
OXFORD.